|
Size: 3012
Comment:
|
Size: 5951
Comment:
|
| Deletions are marked like this. | Additions are marked like this. |
| Line 2: | Line 2: |
| goto [:interact:interact main page] [[TableOfContents]] |
goto [[interact|interact main page]] <<TableOfContents>> |
| Line 40: | Line 40: |
| attachment:binomial.png == Fractals Generated By Digit Sets and Dilation Matrices == |
{{attachment:binomial.png}} == Fractals Generated By Digit Sets and Dilation Matrices (Sage Days 9 - Avra Laarakker) == |
| Line 65: | Line 65: |
| attachment:1.png | {{attachment:1.png}} |
| Line 89: | Line 89: |
| attachment:2.png | {{attachment:2.png}} |
| Line 113: | Line 113: |
| G += sum([text(str(v),v) for v in Dn(D,A,k)]) | G += sum([text3d(str(v),v) for v in Dn(D,A,k)]) |
| Line 117: | Line 117: |
| attachment:3.png attachment:4.png |
{{attachment:3.png}} {{attachment:4.png}} |
| Line 121: | Line 122: |
== Exploring Mandelbrot == Pablo Angulo {{{ %cython import numpy as np def mandelbrot_cython(float x0,float x1,float y0,float y1,int N=200, int L=50, float R=3): '''returns an array NxN to be plotted with matrix_plot ''' cdef int h, i, k m= np.zeros([N,N], dtype=np.int) for i in range(N): for k in range(N): c=complex(x0+i*(x1-x0)/N, y0+k*(y1-y0)/N) z=complex(0,0) h=0 while (h<L) and (abs(z)<R): z=z*z+c h+=1 m[i,k]=h return m }}} {{{ @interact def showme_mandelbrot(x0=-2, y0=-1.5, side=3.0,N=(100*i for i in range(1,11)), L=(20*i for i in range(1,11)) ): time m=mandelbrot_cython(x0 ,x0 + side ,y0 ,y0 + side , N, L ) time show(matrix_plot(m)) }}} {{attachment:mandelbrot_cython.png}} == Mandelbrot & Julia Interact with variable exponent == published notebook: [[http://sagenb.org/pub/1299/]] === Mandelbrot === by Harald Schilly {{{ @interact def mandel_plot(expo = slider(-10,10,0.1,2), \ formula = list(['mandel','ff']),\ iterations=slider(1,100,1,30), \ zoom_x = range_slider(-2,2,0.01,(-2,1)), \ zoom_y = range_slider(-2,2,0.01,(-1.5,1.5))): var('z c') f(z,c) = z^expo + c ff_m = fast_callable(f, vars=[z,c], domain=CDF) # messing around with fast_callable for i in range(int(iterations)/3): f(z,c) = f(z,c)^expo+c ff = fast_callable(f, vars=[z,c], domain=CDF) def mandel(z): c = z for i in range(iterations): z = ff_m(z,c) if abs(z) > 2: return z return z print 'z <- z^%s + c' % expo # calling ff three times, otherwise it fast_callable exceeds a recursion limit if formula is 'ff': func = lambda z: ff(ff(ff(z,z),z),z) elif formula is 'mandel': func = mandel complex_plot(func, zoom_x,zoom_y, plot_points=200, dpi=150).show(frame=True, aspect_ratio=1) }}} === Julia === by Harald Schilly {{{ @interact def julia_plot(expo = slider(-10,10,0.1,2), \ iterations=slider(1,100,1,30), \ c_real = slider(-2,2,0.01,0.5), \ c_imag = slider(-2,2,0.01,0.5), \ zoom_x = range_slider(-2,2,0.01,(-1.5,1.5)), \ zoom_y = range_slider(-2,2,0.01,(-1.5,1.5))): var('z') I = CDF.gen() f(z) = z^expo + c_real + c_imag*I ff_j = fast_callable(f, vars=[z], domain=CDF) def julia(z): for i in range(iterations): z = ff_j(z) if abs(z) > 2: return z return z print 'z <- z^%s + (%s+%s*I)' % (expo, c_real, c_imag) complex_plot(julia, zoom_x,zoom_y, plot_points=200, dpi=150).show(frame=True, aspect_ratio=1) }}} {{{ julia_plot(-7,30,0.5,0.5,(-1.5,1.5), (-1.5,1.5)) }}} |
Sage Interactions - Fractal
goto interact main page
Contents
-
Sage Interactions - Fractal
- Mandelbrot's Fractal Binomial Distribution
- Fractals Generated By Digit Sets and Dilation Matrices (Sage Days 9 - Avra Laarakker)
- Attempt at Generating all integer vectors with Digits D and Matrix A (How about vector([0,-1])?)
- Demonstrating that the Twin Dragon Matrix is likely to yield a Tiling of a Compact Interval of R^2 as k->infinity (It does!)
- Now in 3d
- Exploring Mandelbrot
- Mandelbrot & Julia Interact with variable exponent
Mandelbrot's Fractal Binomial Distribution
def muk_plot(m0,k):
"""
Return a plot of the binomial fractal measure mu_k
associated to m0, 1-m0, and k.
"""
k = int(k)
m0 = float(m0)
m1 = float(1 - m0)
assert m0 > 0 and m1 > 0, "both must be positive"
v = [(0,0)]
t = 0
two = int(2)
delta = float(1/2^k)
multiplier = float(2^k)
for i in [0..2^k-1]:
t = i * delta
phi1 = i.str(two).count("1")
phi0 = k - phi1
y = m0^(phi0)*m1^(phi1)*multiplier
v.append((t,y))
v.append((t+delta,y))
return v
html("<h1>Mandelbrot's Fractal Binomial Measure</h1>")
@interact
def _(mu0=(0.3,(0.0001,0.999)), k=(3,(1..14)), thickness=(1.0,(0.1,0.2,..,1.0))):
v = muk_plot(mu0,k)
line(v,thickness=thickness).show(xmin=0.5, xmax=0.5, ymin=0, figsize=[8,3])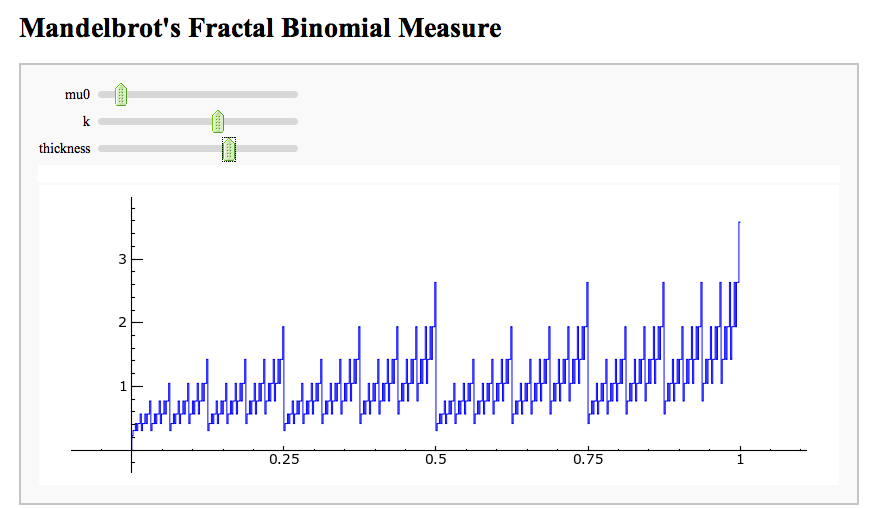
Fractals Generated By Digit Sets and Dilation Matrices (Sage Days 9 - Avra Laarakker)
Attempt at Generating all integer vectors with Digits D and Matrix A (How about vector([0,-1])?)
A = matrix([[1,1],[-1,1]])
D = [vector([0,0]), vector([1,0])]
@interact
def f(A = matrix([[1,1],[-1,1]]), D = '[[0,0],[1,0]]', k=(3..17)):
print "Det = ", A.det()
D = matrix(eval(D)).rows()
def Dn(k):
ans = []
for d in Tuples(D, k):
s = sum(A^n*d[n] for n in range(k))
ans.append(s)
return ans
G = points([v.list() for v in Dn(k)])
show(G, frame=True, axes=False)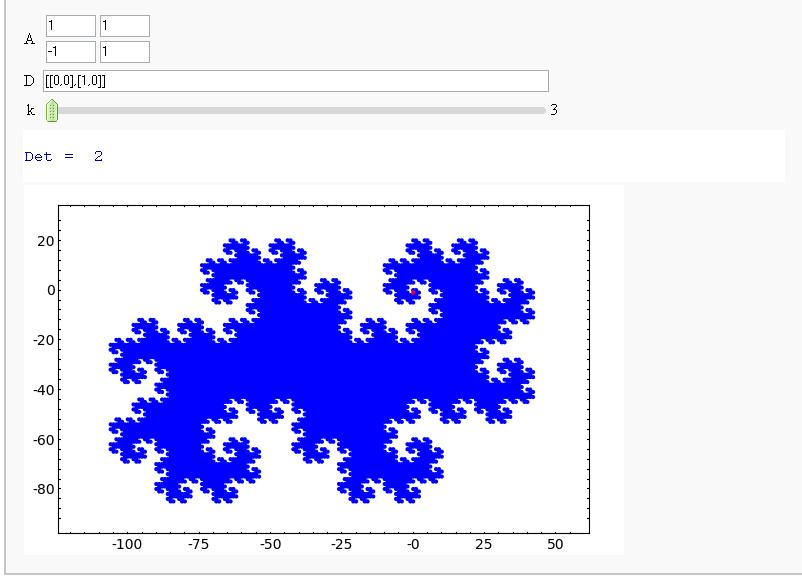
Demonstrating that the Twin Dragon Matrix is likely to yield a Tiling of a Compact Interval of R^2 as k->infinity (It does!)
A = matrix([[1,1],[-1,1]])
D = [vector([0,0]), vector([1,0])]
@interact
def f(A = matrix([[1,1],[-1,1]]), D = '[[0,0],[1,0]]', k=(3..17)):
print "Det = ", A.det()
D = matrix(eval(D)).rows()
def Dn(k):
ans = []
for d in Tuples(D, k):
s = sum(A^(-n)*d[n] for n in range(k))
ans.append(s)
return ans
G = points([v.list() for v in Dn(k)])
show(G, frame=True, axes=False)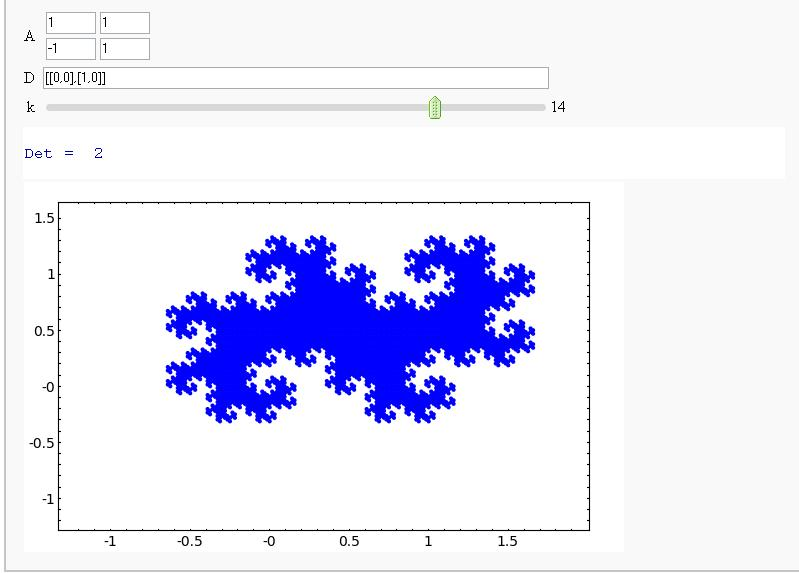
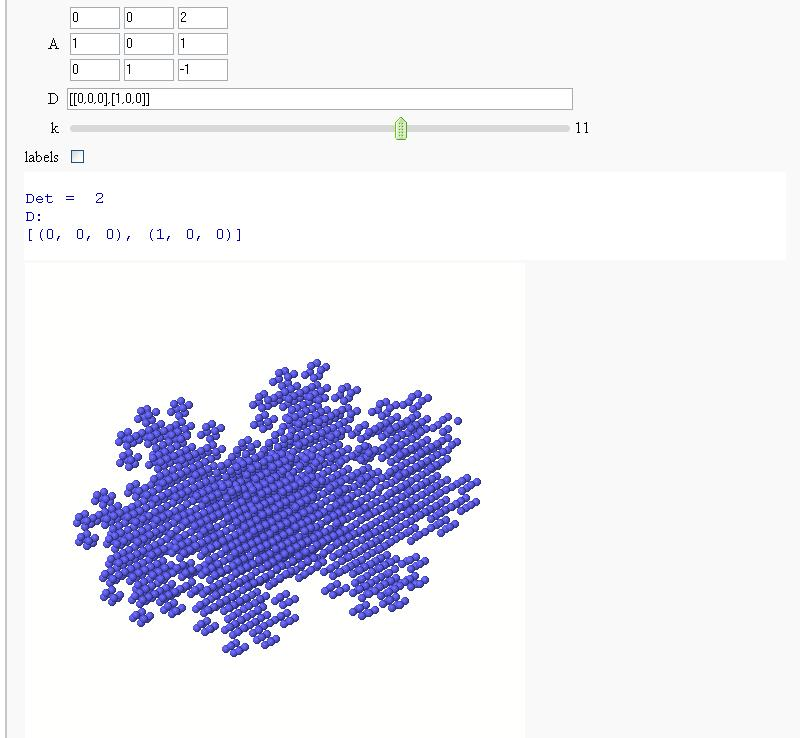
Now in 3d
A = matrix([[0,0,2],[1,0,1],[0,1,-1]])
D = '[[0,0,0],[1,0,0]]'
def Dn(D,A,k):
ans = []
for d in Tuples(D, k):
s = sum(A^n*d[n] for n in range(k))
ans.append(s)
return ans
@interact
def f(A = matrix([[0,0,2],[1,0,1],[0,1,-1]]), D = '[[0,0,0],[1,0,0]]', k=(3..15), labels=True):
print "Det = ", A.det()
D = matrix(eval(D)).rows()
print "D:"
print D
G = point3d([v.list() for v in Dn(D,A,k)], size=8)#, opacity=.85)
if labels:
G += sum([text3d(str(v),v) for v in Dn(D,A,k)])
show(G, axes=False, frame=False)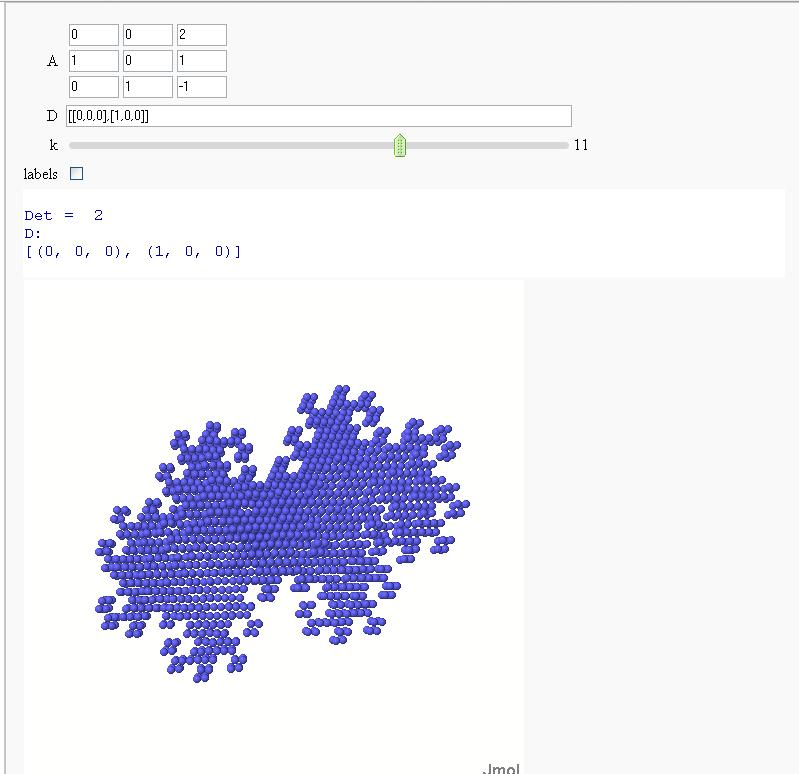
Exploring Mandelbrot
Pablo Angulo
%cython
import numpy as np
def mandelbrot_cython(float x0,float x1,float y0,float y1,int N=200, int L=50, float R=3):
'''returns an array NxN to be plotted with matrix_plot
'''
cdef int h, i, k
m= np.zeros([N,N], dtype=np.int)
for i in range(N):
for k in range(N):
c=complex(x0+i*(x1-x0)/N, y0+k*(y1-y0)/N)
z=complex(0,0)
h=0
while (h<L) and (abs(z)<R):
z=z*z+c
h+=1
m[i,k]=h
return m@interact
def showme_mandelbrot(x0=-2, y0=-1.5, side=3.0,N=(100*i for i in range(1,11)), L=(20*i for i in range(1,11)) ):
time m=mandelbrot_cython(x0 ,x0 + side ,y0 ,y0 + side , N, L )
time show(matrix_plot(m))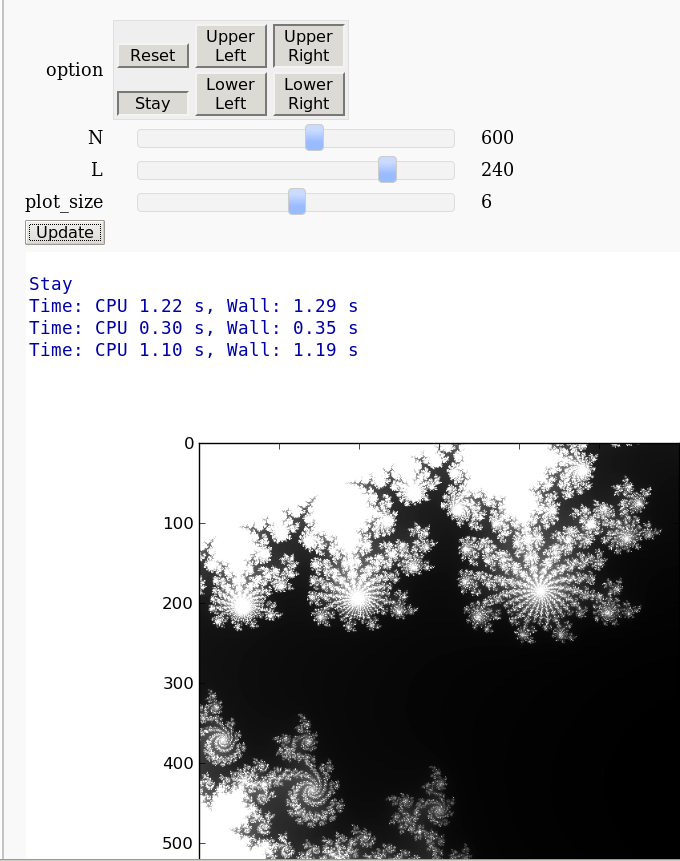
Mandelbrot & Julia Interact with variable exponent
published notebook: http://sagenb.org/pub/1299/
Mandelbrot
by Harald Schilly
@interact
def mandel_plot(expo = slider(-10,10,0.1,2), \
formula = list(['mandel','ff']),\
iterations=slider(1,100,1,30), \
zoom_x = range_slider(-2,2,0.01,(-2,1)), \
zoom_y = range_slider(-2,2,0.01,(-1.5,1.5))):
var('z c')
f(z,c) = z^expo + c
ff_m = fast_callable(f, vars=[z,c], domain=CDF)
# messing around with fast_callable
for i in range(int(iterations)/3):
f(z,c) = f(z,c)^expo+c
ff = fast_callable(f, vars=[z,c], domain=CDF)
def mandel(z):
c = z
for i in range(iterations):
z = ff_m(z,c)
if abs(z) > 2:
return z
return z
print 'z <- z^%s + c' % expo
# calling ff three times, otherwise it fast_callable exceeds a recursion limit
if formula is 'ff':
func = lambda z: ff(ff(ff(z,z),z),z)
elif formula is 'mandel':
func = mandel
complex_plot(func, zoom_x,zoom_y, plot_points=200, dpi=150).show(frame=True, aspect_ratio=1)
Julia
by Harald Schilly
@interact
def julia_plot(expo = slider(-10,10,0.1,2), \
iterations=slider(1,100,1,30), \
c_real = slider(-2,2,0.01,0.5), \
c_imag = slider(-2,2,0.01,0.5), \
zoom_x = range_slider(-2,2,0.01,(-1.5,1.5)), \
zoom_y = range_slider(-2,2,0.01,(-1.5,1.5))):
var('z')
I = CDF.gen()
f(z) = z^expo + c_real + c_imag*I
ff_j = fast_callable(f, vars=[z], domain=CDF)
def julia(z):
for i in range(iterations):
z = ff_j(z)
if abs(z) > 2:
return z
return z
print 'z <- z^%s + (%s+%s*I)' % (expo, c_real, c_imag)
complex_plot(julia, zoom_x,zoom_y, plot_points=200, dpi=150).show(frame=True, aspect_ratio=1)julia_plot(-7,30,0.5,0.5,(-1.5,1.5), (-1.5,1.5))
